Every discovery and invention aids in unravelling mysteries that has been puzzling mankind for ages. But, at what cost, entangling more knots to the already existing unsolved conundrum. Talking of loops, let’s start with a classical mathematical joke.
“Why did the chicken cross the mobius strip?
To get to the same side.”
If you got the joke, then you are already familiar with one of the bizarre mathematical objects, the mobius strip; also known as the mobius loop or the mobius band. In case if you don’t get the joke, worry not; after reading this article, you will.
Is it possible to get to the same side by crossing an edge? If your answer is no, then read the joke again. The chicken crossed to get to the same side. Then by crossing an edge, the chicken must have got to the other side. Every surface has two sides, inside and the outside. But how did it end up at the same side? Mobius strip, a non-Euclidean object is one of the exceptions to the rule. The study of mobius strip led foundation to the field Topology.
Before getting to know more about Mobius strip, let’s have a quick recap of certain terms.

Vertex – A point where two or more lines, curves or edges meet in a graph.
Edge – A type of line segment (abstract object) that connects two vertices of a graph.
Surface – A generalization of a plane whose curvature is not necessarily zero i.e. not definitely flat.
Surface with boundary – A topological space obtained by identifying edges. Their vertices are called boundary vertices.
Orientable surface – A surface S in the Euclidean space is said to be orientable if a 2d figure can’t be moved around the surface and back to where it started so that it looks like its own mirror image.
In other words, a surface is non-orientable if you can walk along some path and come back to where you started but reflected.
WHAT IS A MOBIUS STRIP?

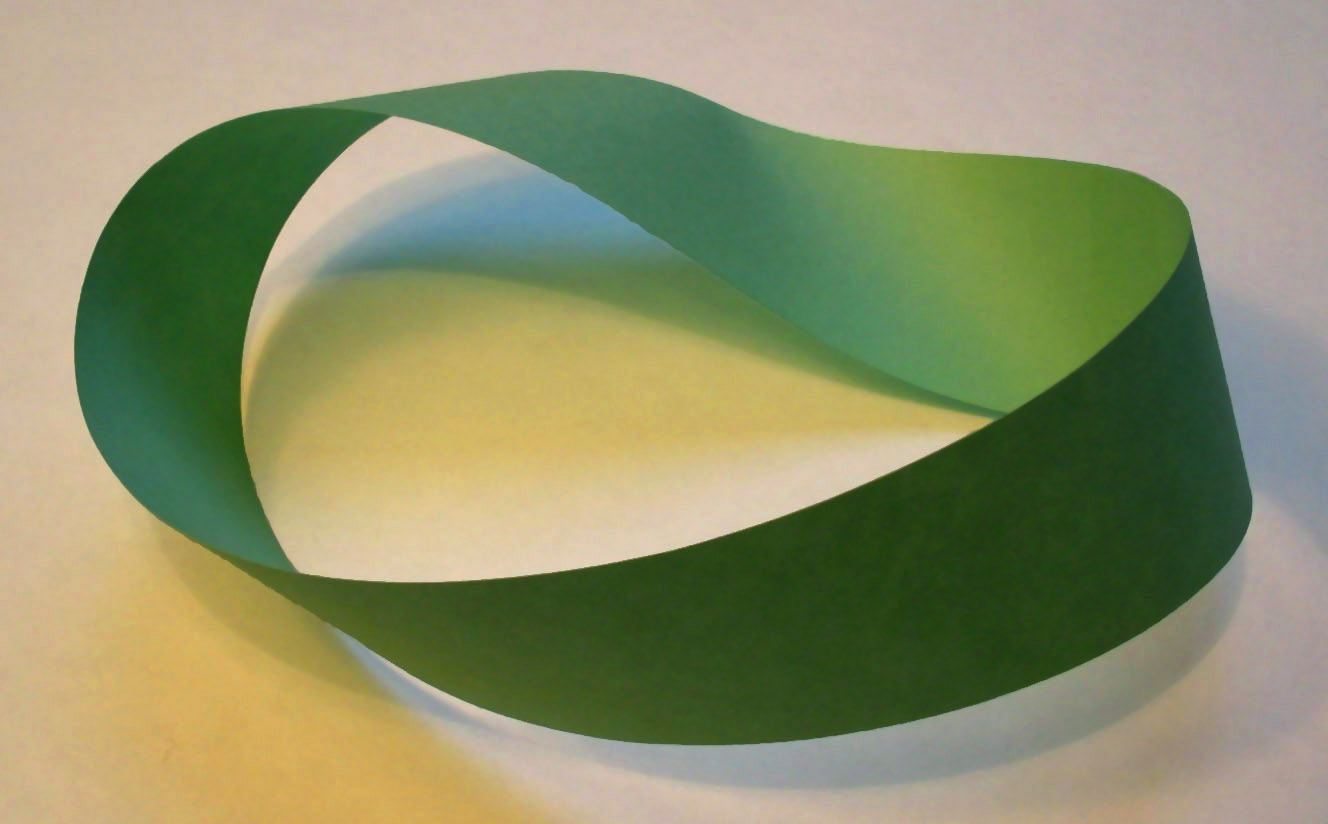
Mobius strip is a two-dimensional non orientable surface that has only one side when embedded in three-dimension. It is an example of surface with boundary. It may sound absurd at first. But you can visualize it merely by drawing a line through the curve. Start from a point, draw through the curve, without crossing an edge you can observe that you can reach the other side of the curve. It has only one side and one edge. The shape of mobius strip resemble that of infinity.
To trace the edge of a mobius strip, starting from a point, draw along its length (say l). When your line measures a length of l units, you would be at a point exactly opposite from the starting point (directly below). Continue for another l units, then you will reach the starting point. Thus you get to the other side without crossing an edge. The traced line runs on both sides of the paper.

HISTORY OF MOBIUS STRIP:
Mobius strip was discovered by August Ferdinand Mobius, a German mathematician and an astronomer of 19th century, a student of Carl Fredrich Gauss. Fun fact is, Johann Benedict Listing, another German mathematician independently discovered the same, few months prior to Mobius. But it ended up being named after Mobius. Mobius has a lot of stuffs named after him. To people who think it’s a modern invention, you are mistaken. It is seen in the Roman mosaic of 3rd century dating 1600 years back. The most ancient imagery of the Mobius strip to be known is Aeon mosaic.
MODELLING A MOBIUS STRIP
Mobius strip is not a complex, unimaginable, far-fetched object. All you need is a strip of paper and glue to make it. Take a strip of paper whose length is much greater than its width (say 10 times). Twist an end by 180° and glue with the other end. Congratulations, you’ve a mobius band with you. You can make a mobius band with any number of 180° twists but odd. Odd number of twists gives you a band that has no inside or outside. Even numbered yields a band that has both inside and outside.
A torus (donut-shape) can be turned into a mobius strip with an even number of half twists and a Klein bottle can also be used to form mobius strip (not one, but two) by cutting into half, along its length. Two strips, on top of one another, each with a half twist (180°) give a single loop with four twists when disentangled.
MATH BEHIND THE LEGEND:
When the mobius strip itself is non-orientable, any non-orientable surface embedded in space R3 is an orientable surface with a positive number of Mobius strips attached to it. What we have made with a strip of paper is only a model of Mobius strip; when in reality, Mobius strip is a two dimensional manifold. How to find the surface area of a mobius strip? It depends on how we started with the strip. Since we started with a strip of paper, we can obtain the area of the mobius strip by finding the area of the strip of paper i.e. take the product of length and breadth and multiply by two since the paper has two sides, we add the surface area of both the sides. Or you can trace the length of the mobius strip and multiply with its breadth. And you have the area now. Otherwise you can obtain the surface area by integrating the parametric form of the equation.
REAL WORLD APPLICATIONS:
Mobius strip has been an inspiration to many children toys. It has inspired writers a lot, that there are movies and songs based on this. Several logos have been influenced by mobius strip not to mention Google drive and recycling logo. Adobe reader’s logo could be added to the list as well. Mobius band model is used in the conveyor belts to undergo uniform wear and tear on both sides. Recording tapes and printer ribbons follow the same as well which aids in printer ribbons utilized to the maximum. Recording tapes use it to double the play time. Models based on mobius strip has been patented for usage in several electrical components including resistors, molecular motors and graphene structures (Graphene mobius strips).
They are not just man made objects. Several natural phenomena endow the properties mobius strip. For example, the trajectory of charged particles trapped in the magnetic field of the Earth, as in the van Allen belts that describes a Mobius strip before its move to become chaotic. Few of the proteins’ structural composition is same as the Mobius strip, even their biological activity depends on this morphology. Lemiscate (shape of symbol infinity) is also similar to Mobius band.
Activities based on mobius strip could motivate children to learn math in a fun way, surging their love for mathematics. Advanced mathematical topics like topology could be introduced to kids with these kinds of fun experiments, opening up new world full of opportunities for them. Cutting along the length of the strip at a distance of 1/3rd of the width results in a mobius strip and a normal band. If you cut along the length exactly at the center, you get two intertwined mobius strips. Cutting along the length at different places, gives different results for different number of twists. Try cutting the strip at different places along the length and share what interesting stuffs you find.
You may also like- Gabriel’s horn: Perceiving the infinite in the Finite!








